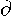 mAn
+ Gnrm Ar
mAn
+ Gnrm ArFRACTAL SPACE-TIME AND MICROPHYSICS.
Towards a Theory of Scale Relativity.
Laurent Nottale
© World Scientific (Singapore, New Jersey, London, 1993)
Chapter 1
GENERAL INTRODUCTION
The essential question that is addressed in this book is the problem of scales in Nature. This is not a recent question. From Plato, Euclid, and Aristotle, to Leibniz, Laplace, and Poincaré, many philosophers, mathematicians and physicists have thought over scales and their transformations, dilations and contractions. What determines the universal scales in Nature? What is the origin of the elementary particles scales, of the unification and symmetry breaking scales, of the large scale structures in the Universe? Not only are fundamental or characteristic scales observed to occur in the world, but physical laws may in some situations depend themselves on scale: this leads us to the concepts of scaling and of scale invariance.
As reminded in Chapter 2, this scale dependence may in some cases be very fundamental: hence in quantum mechanics the results of measurements explicitly depend on the resolution of the measurement apparatus, as described by the Heisenberg relations; in cosmology, it is the whole set of interdistances between the objects of the Universe that depends on a time varying universal scale factor (this is the expansion of the Universe). Moreover, scale laws and scaling behaviours are encountered in many situations, at small scales (microphysics), large scales (extragalactic astrophysics and cosmology) and intermediate scales (complex self-organized systems), but most of the time such laws are found in an empirical way, since we still lack a fundamental theory allowing us to understand them from fundamental principles.
Our proposal is that such a fundamental principle upon which a theory of scale laws may be founded is the principle of relativity itself. But, by `principle of relativity' we mean something more general than its application to particular laws: we actually mean a universal method of thought. Following Einstein, we shall express it by postulating that the laws of Nature must be such that they apply to reference systems whatever their state. The present theory of relativity, after the work of Galileo, Poincaré and Einstein, results from the application of this principle to space and time coordinate systems and to their state of position (origin and axis orientation) and of motion (which may be eventually included into axis orientation in space-time).
We have suggested that the principle of relativity also applies to laws of scale. The present book is an account of this proposal and of its possible first implications. Taking advantage of the relative character of every length and time scales in Nature, we define the resolution of measurements (more generally, the characteristic scale of a given phenomenon) as the state of scale of the reference system. This allows us to set a principle of scale relativity, according to which the laws of physics must be such that they apply to coordinate systems whatever their state of scale, whose mathematical translation is the requirement of scale covariance of the equations of physics. While the classical domain is apparently unchanged by such an analysis, its fundamental laws being scale independent (but situations where dynamical chaos occurs may call for a reopening of the question, see Chapter 7), there are two fundamental scale-dependent domains on which this extension of the principle of relativity sheds new light, namely quantum physics and cosmology.
In order to describe physical laws complying to this principle, one needs some mathematical tools capable of achieving such a fundamental and explicit dependence of physics on scale in their very definition. There is one geometrical concept that immediately comes to mind in this respect, Mandelbrot's so-called fractals, a word that names objects, sets and functions whose forms are extremely irregular and fragmented on all scales. But on the other hand, physical laws that are explicitly scale dependent have already been introduced in physics from algebraic methods, mainly by the development of the renormalization group in Wilson's many scales of length approach. Both tools are considered and developed in the present book; their connection is revealed by the remark that the standard measures on fractals (based on their topological dimension, such as length, area, volume...) are solutions of renormalization group-like equations.
Chapter 3 is devoted to a reminder about fractals and to a first attempt at going beyond fractal objects seen as embedded into Euclidean space, in order to reach an intrinsic definition of the concept of fractal space (more generally space-time). We introduce new mathematical tools for physics, such as non-standard analysis and scale-dependent fractal functions, that allow us to deal with the non-differentiability and infinities characterizing fractal geometry.
Then in Chapter 4 we consider the behaviour of quantum mechanical paths
in the light of the fractal tool. As first demonstrated by Feynman, typical
quantum mechanical trajectories are characterized by their non-differentiability
and their fractal structure. It is demonstrated that the Heisenberg relations
can be translated in terms of a fractal dimension of all four space-time
coordinates jumping from D=2 in the quantum and quantum relativistic
domain to D=1 (i.e. non-fractal) in the classical domain, the transition
being identified as the de Broglie scale lm=
h /pm.
The case for the fractal and non-differentiable structure of the quantum space-time is argued further in Chapter 5. We demonstrate that generalized Heisenberg relations may be deduced from the conjectured fractal structure, and that a perfect point following a D=2 fractal trajectory owns an intrinsic angular momentum that is identified to quantum spin. The wave-particle duality is understood in terms of infinite families of equiprobable geodesics on a fractal space-time. We then suggest a new formulation of stochastic quantum mechanics which allows us to identify Schrödinger's equation with Newton's fundamental equation of dynamics written in a non-differentiable space.
In Chapter 6, we apply the principle of scale relativity to the renormalization group approach in microphysics. We first reformulate in terms of renormalization group equations the results that were described in previous Chapters in terms of fractal structures. This allows us to demonstrate that, in its present form, the renormalization group for the quantum space-time owns a Galilean-like mathematical structure, while the general solution to the `special relativity problem' (i.e., find the linear transformations that satisfy Galileo's principle of relativity) may be shown to be the Lorentz group.
Then the introduction of a Lorentz-like renormalization group,
in conjunction with the breaking of the scale relativity symmetry at the
de Broglie scale (transition from scale-dependence to scale-independence),
leads to a demonstration of the existence of a universal, lower, limiting
scale in Nature, that is invariant under dilatations and plays
the same role for scale as that played by the velocity of light c for
motion. It is identified with the Planck scale (Lp
= (hG/c3)1/2 ~
1.6 10-35 m; Tp
= Lp
/c = (hG/c5)1/2
~ 5.4 10-44 s), which,
in such a new framework, owns all the properties of the previous perfect
zero point. The de Broglie and Heisenberg relations are generalized: energy-momentum
now tends to infinity when the length-time scale tends to the Planck scale.
Although the largest effects of such a new structure are expected at the
Planck scale, at which space-time would become totally degenerated, it
also has observable consequences in the domain of energy presently accessible
to experiment.
The elementary charges and masses (self-energies), which were divergent in the current quantum theory, become finite in the new theory. "Bare" charges are now well-defined: arguments are presented which lead us to conclude that the value of the common elementary bare charge is 1 / 2p (in dimensionless units). The values of the coupling constants observed at low energy may then be predicted from their renormalization group equations.
New universal scales naturally emerge in this `scale covariant' frame. Namely we predict one scale that agrees very closely with the observed electroweak symmetry breaking scale and a second scale whose ratio with the Planck scale is in good agreement with the `Grand Unification' scale.
The four fundamental couplings, electroweak U(1) and SU(2), colour SU(3), and gravitational, converge in the new framework towards the same scale, which is nothing but the Planck mass / energy scale. We conclude this chapter with some proposals concerning the origin of the weak boson masses and the nature of the electric charge.
Chapter 7 tentatively applies the principle of scale relativity to cosmology. We first consider the consequences for the standard Big Bang of the new structures suggested in microphysics; then the implication of scale relativity for observational cosmology are analysed. As in microphysics, cosmology is characterized by a fundamental scale dependence of physical laws, as exemplified in the expansion of the Universe. The situation is symmetrical to the microphysical one, and we tentatively reach similar conclusions: there exists a universal, upper limiting scale L in Nature which is invariant under dilatations. We identify this scale as yielding the true nature of the cosmological constant L, L = L- 1/2. In such a perspective, Mach's principle and Dirac's large numbers hypothesis can be reactualized.
Then we jump to a different (but related) subject, by showing that the methods of non-differentiable geometry that were developed in Chapter 5 can also be applied to situations involving dynamical chaos. This is exemplified by a reconsideration of the problem of the distribution of planets in the Solar System. We finally conclude by a prospect about future possible developments of this new field.
Chapter 2
RELATIVITY
AND
QUANTUM PHYSICS
2.1. On the Present State of Fundamental Physics.
The laws of physics are presently described in the framework of two main theories, namely the theory of relativity (special1-2 and general,3 which include classical mechanics) and quantum mechanics4-6 (developed into quantum field theories). Both edifices are extremely efficient and precise in their predictions; the constraints imposed by special relativity have even been incorporated in a relativistic quantum theory. But these two theories are founded on completely different grounds, even contradictory in appearance, and make use of a completely different mathematical apparatus.
General relativity is a theory based on fundamental physical principles, namely the principles of general covariance and of equivalence. Its mathematical tools come as natural achievements of these principles. On the contrary quantum mechanics is, at present, an axiomatic theory. It is founded on purely mathematical rules which, up to now, are not understood in terms of a more basic mechanism.
This leads to a strong dichotomy in physics: two apparently opposite worlds cohabit, the classical and the quantum. In particular gravitation, so clearly and accurately described by Einstein's theory of general relativity,3 has escaped up to now any admissible description in terms of the quantum field-particle approach. Conversely, our understanding of the electromagnetic, weak and strong fields has made huge progress in the framework of quantum gauge theories,7-9 while all classical attempts to unification (e.g., of gravitation and electromagnetism) have ended in failure.
These and other signs indicate, in our opinion, that physics is still in infancy. Several great problems, maybe the most fundamental ones, are still completely open. There is at present no theory able to make predictions about the two "tails" of the physical world, namely elementarity and globality, i.e., at the smallest and largest time scales and length scales.
At small scales, the "standard model" of elementary particles, based on quantum chromodynamics and electroweakdynamics, is able to include in its framework the observed structure of elementary particles and coupling constants (i.e., charges). But it seems, up to now, unable to predict on purely theoretical grounds either the number of elementary particles, or their masses, nor the values of the fundamental couplings. This failure is certainly related to the main failure of electrodynamics (classical and quantum): the divergence of self-energy and charge at infinite energy.10 Renormalization11-13 was only a partial solution to the problem. By replacing in calculations the theoretical infinite charges and masses by the observed ones, it allowed physicists to predict with high precision all the other physical quantities of interest. But the problem of masses and charges was left open.
At the other end, that of very large scales, even though the current cosmological theory has known great successes, one must not forget that general relativity, being a purely local theory (its fundamental tool, the metrics element, is differential), tells us nothing about the global topology of the Universe.14 This is, with the problem of sources of gravitation (why does inertia curve space-time?), one of the limiting domains where general relativity is an incomplete theory, as recognized by Einstein himself:15 an indication of this incompleteness may be its inability to include Mach's principle, except in some particular models, while observations seem to imply that it is effectively achieved by Nature (see Secs. 5.11 and 7.1).
The intermediate classical world is not devoid of open fundamental problems. Recent years have known an impressive burst in the study of dynamical chaos.16-18 Chaos is defined as a high sensibility on initial conditions which leads to rapid divergence (e.g. exponential) of initially close trajectories, then to a complete loss of predictability on large time scales. Chaos is encountered in equations which look quite deterministic, in a large number of different domains like chemistry, fluid mechanics and turbulence, economics, population dynamics, celestial mechanics, meteorology... The challenge of chaos is that structures are very often observed in domains where chaos has developed, while ordinary methods fail to make prediction because of the presence of chaos itself. The understanding of how "order" (better: "organization") emerges from chaos is the key for the foundation of a future (still not existing) science of classical complexity. This fundamental problem will be addressed in Secs. 3.2, 5.6, 5.7 and 7.2.
We shall attempt to convince the reader that these questions, in the quantum, cosmological and classical complexity domains, may actually be of a similar nature. They all turn around the problem of scales, and may be traced back to a still unanswered very fundamental question: what determines the fundamental scales in Nature? A theory of scale is needed in physics. We shall propose here that Einstein's principle of relativity applies not only to laws of motion but also to laws of scale, and thus can be used as a basic stone for founding such a theory. But let us first briefly describe the present status of the theories of relativity and of quantum mechanics.
The theory of relativity.
Galilean relativity, Einstein's special and general theories of relativity are successive attempts to make possible the expression of physical laws in more and more general coordinate systems. Let us recall Einstein's statement of the principle of general relativity:3 "the laws of physics must be of such a nature that they apply to systems of reference in any kind of motion". Remark that, under this form, this principle is, strictly speaking, a principle of the relativity of motion. This principle is particularly remarkable by the combination of its simplicity and its extraordinary power for deriving the most fundamental constraints which govern the physical world. Take Galileo's statement of the principle: "motion is like nothing". At first sight it may look like a trivial statement. But the explicit expression of this principle actually imposes strong universal constraints about the possible forms that physical laws can take. Since motion cannot be detected by purely local experiments, only some particular laws of transformation between inertial systems are admissible. This leads to the classical laws of Galilean physics and, adding the postulate of the invariance of some velocity c, to Einstein-Poincaré-Lorentz special relativity. Moreover we shall demonstrate in Sec. 6.4 that this additional postulate is not necessary for deriving the Lorentz transform: i.e., the Lorentz transform may be shown to be the general transformation which achieves the principle of special relativity in its Galilean form.
Special relativity leads to the constraint that no velocity can exceed some universal velocity c, which may subsequently be shown to be the velocity of particles of null mass, in particular that of light.19 Recall that the Minkowskian space-time is characterized by the invariant
ds2 = c2dt2 - (dx2 + dy2 + dz2) ,
under any change of inertial coordinate system. Then it was one of Mach's main contributions to the evolution of physics to insist on the relativity of all motions, not only of inertial ones. From general covariance and the principle of equivalence, Einstein constructed the theory of general relativity, whose equations are constraints on the possible curvatures of space-time. Einstein's equations
Rmn - (1/2) R gmn - L gmn = c Tmn (2.1)
are the most general simplest equations which are invariant under any continuous and differentiable transformation of coordinate systems.
For a full account of the theory, we send the reader to textbooks as those by Misner, Thorne and Wheeler22 or Weinberg.55 Let us only briefly recall here that, in these equations, the gmn's are tensorial metric potentials which generalize the Newtonian gravitational scalar potential. The general relativistic invariant reads, in terms of Einstein's convention of summation on identical lower and upper indices
ds2 = gmn dxm dxn , (m,n = 0 to 3).
In general relativity, the curvature of space-time implies that the variation of physical beings (such as vectors or tensors) for infinitesimal coordinate variations depends also on space-time itself. This is expressed by the covariant derivative
DmAn
= 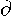 mAn
+ Gnrm Ar
mAn
+ Gnrm Ar
which generalizes the partial derivatives. In this expression, the effect of space-time (i.e., of gravitation) is described by the Christoffel symbols
Grmn = (1/2)
grl ( 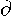 n
glm +
n
glm + 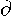 m
gln -
m
gln -
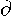 l gmn
) ,
l gmn
) ,
which play the role of the gravitational field. The covariant derivatives do not commute, so that their commutator leads to the appearance of a four- indices tensor, the so-called Riemann tensor Rlmnr :
(Dm Dn - Dn Dm ) Ar = Rlrnm Al .
Contraction of the Riemann tensor yields the Ricci tensor Rmn = glrRlmrn :
Rmn = 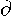 rGrmn
-
rGrmn
- 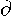 nGrmr + Grmn
Glrl - Grml Glnr
,
nGrmr + Grmn
Glrl - Grml Glnr
,
while the quantity R=gmnRmn is the scalar curvature. Einstein's equations state that the energy-momentum tensor Tmn is equal, up to the constant c = 8pG/c4, to the geometric Einstein tensor given in the first member of (2.1), in which L is the cosmological constant. The Einstein tensor and the energy-momentum tensor are conservative in the covariant sense. Einstein's equivalence principle of gravitation and inertia is expressed by the fact that one may always find a coordinate system in which the metric is locally Minkowskian, and that in such a system the equation of motion of a free particle is that of inertial motion, Dum = 0, where um is the four-velocity of the particle. Written in any coordinate system, this equation becomes the geodesics equation
d2xm/ds2 + Gmnr (dxn/ds) (dxr/ds) = 0 .
Note that the principle of relativity, in Einstein's formulation, applies to the "laws of Nature", which Einstein carefully distinguishes from the equations of physics. Laws of Nature are assumed to exist independently from the physicist (this is the first underlying working postulate of any "philosophy of nature") while equations of physics are the mathematical expression of our own (always perfectible) attempts at reaching them. The mathematical translation of the principle of general relativity is Einstein's principle of general covariance:3 "the general laws of nature are to be expressed by equations which hold good for all systems of coordinates, that is, are covariant with respect to any substitutions whatever".
The evolution of the principle of relativity is intrinsically linked to evolution of the concept of space-time. Any interrogation about the physics involved in the transformation of reference frames runs into an interrogation about space-time. Reversely, asking questions about space-time leads one to question relativity. In Galilean relativity, space and time are assumed to be absolute and independent concepts. The special theory1-2 renounces such views, and introduces the concept of space-time.20 But the Minkowskian space-time is still absolute, while the analysis by Mach, then by Einstein,3 clearly shows the general covariance requirement to be inconsistent with the idea of a privileged space or space-time. This leads to the space-time of the general theory which depends on the material and energetic content of the universe.
The way through which space-time properties are related to matter properties is instructive (by "matter" we mean matter and energy). It consists in attributing to space-time those properties of matter which are universal. It is the universality of the Lorentz transform, which applies not only to electromagnetic waves but also to any kind of massive particle or system, that allows the introduction of the Minkowskian space-time. In general relativity, the universal property of matter pointed out by Einstein is the curvature of trajectories. This allows one to understand the crucial role played by the deviation of light rays (and more generally by all effects of gravitation on light) in the construction of the theory21 and in its final acceptance. The universality of the curved nature of trajectories of particles, whether massive or not, leads to attributing the property of curvature to space-time itself. Then in the curved space-time, free particles follow geodesical lines, in agreement with the equivalence principle.
The power of this approach is appreciable when remembering that, if one completely accepts Einstein's geometrical interpretation, the concepts of forces, of potentials and of field disappear for the benefit of the mere curved space-time: from the principle of equivalence, inertia is found back locally in a freely falling reference system, i.e., one which follows a geodesic of the Riemannian space-time. Space-time, as described by the metric potentials, may be considered as a new mathematical tool, even more profound than that of field; from it the notions of force and of potential may be finally recovered, but as approximations. If we push to its logical ends the argument of the vanishing of the field, we get Einstein's radical interpretation of the nature of gravitation, as being nothing but the manifestation of a universal property of the world, the space-time curvature.22
Quantum physics.
At present, quantum mechanics is being based on a completely different approach. Let us briefly recall (and comment) the axioms of the nonrelativistic theory (see e.g. Refs. 23-25):
(i) A physical system is defined by a state function f. Its coordinate realization, the complex wave function y (q,s,t), is often used in the non- relativistic theory. It depends on all classical degrees of freedom, q and t, and on additional purely quantum mechanical degrees of freedom s, such as spin. The probability for the system to have values (q, s) at time t is given by P = |(y (q,s,t) |2, so one may write y in the form y = P1/2 eiq. Following Feynman, one often rather uses the probability amplitude y(a,b) between two space-time events a and b. The extraordinary fact about quantum mechanics is that the full complex probability amplitude, with modulus P1/2 and phase q, is necessary to make correct predictions, while only the square P of the modulus is observed. When an event can occur in two alternative ways the probability amplitude is the sum of the probability amplitudes for each way considered separately:
y = y1 + y2 => P = P1 + P2 + y1 y2 + y2 y1
The two new rectangular terms additional to the classical terms P1 + P2 are at the origin of interferences and more generally of quantum coherence. Conversely, when it is known whether one or the other alternative is actually taken, the composition of probabilities takes the classical form P = P1 + P2 .
(ii) If y1 and y2 are possible states of a system, then y = ay1 +by2 is also a state of the system.
(iii) Physical observables are represented by linear Hermitian operators,
W, acting on the state function. For
example there corresponds to momentum pi the
complex operator - ih
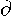 /
/ 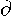 qi . This is another manifestation of the mysterious
character of quantum rules that, to a real momentum, there corresponds
a complex operator acting on the complex probability amplitude.
qi . This is another manifestation of the mysterious
character of quantum rules that, to a real momentum, there corresponds
a complex operator acting on the complex probability amplitude.
(iv) Results of measurements of physical observables are given by any of the eigenvalues of the corresponding operator, W y = wi y.
(v) Any state function can be expanded as y = Sn anyn in an orthonormal basis, and |an|2 records the probability that the system is in the nth eigenstate.
(vi) The time evolution of the system satisfies Schrödinger's equation
H y = i h
(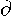 y /
y / 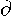 t) ,
t) ,
where the Hamiltonian H is a linear Hermitian operator.
(vii) Immediately after a measurement, the system is in the state given by the first measurement. This seventh axiom ("Von Neumann's axiom") is forgotten in many text books though it is necessary to account for experiments: for example after a spin measurement, the spin remains in the state given by the measurement; just after a measurement of position (at t+dt, dt->0), a particle is in the position given by the measurement. Its absence may give a false impression of quantum mechanics as a theory where precise predictions can never be done, while this depends on the pure or mixed character of the state of the system. It underlies the phenomenon of reduction of the wave packet.26
These axioms have well-known philosophical consequences (or better, they are a self-consistent mathematical transcription of what experiments have told us about the microphysical world: the philosophical consequences originate, in the present thinking frame, from observations). Two realizations of the state function, the coordinate and momentum representations, are particularly relevant in this respect. The position and momentum wave-functions may be derived one from the other by reciprocal Fourier transform. From this comes the Heisenberg inequality
sx sp
>= h / 2 ,
which implies the non-deterministic character of quantum trajectories.
Note also that the solution of Schrödinger's equation for a free particle
leads to the introduction of the de Broglie length and time: The phase
of the wave-function writes q = (p
x - E t) / h,
where p and E are the classical momentum and energy of the
particle. The de Broglie periods, h/p and h/E, correspond
to a phase variation of 2p. Throughout
this book, we shall call "de Broglie length and time" the quantities
l = h / p
; t = h/ E
such that the quantum phase for a free particle writes q = (x /l- t/t). (Recall that historically, the Schrödinger equation was constructed as the equation whose de Broglie wave, obtained earlier, was a solution). The de Broglie scale may be generalized to more complicated systems: it can be identified with the characteristic transition scale occurring in the quantum phase.
It is clear from the above axioms that most of the quantum mystery may be traced back to the mere question: "where does the complex plane of quantum mechanics lie?" We shall in this book propose a solution to this puzzle by showing that a complex plane naturally emerges in space-time from the simplest prescription aimed at describing a non-differentiable space-time; this allows one to obtain Schrödinger's equation as the form taken by the fundamental equation of dynamics written in such a non-differentiable frame (see Chapter 5).
Quantum mechanics is an axiomatic theory rather than a "theory of principle" as relativity. We do not understand the physical origin of these axioms: we only know that they work, i.e., that the theory developed from them has a high predictive power and is remarkably precise. The mathematical beings of the present quantum theory are defined in an abstract space of state, so that the part played by the standard space-time has apparently been deeply decreased. Quantum physics in its present form describes rather the intrinsic properties of microphysical objects embedded in a space-time which is a priori assumed to be Euclidean or Minkowskian. Though it has already been suggested that the structure of the microphysics space-time could be foamy or gruyere cheese-like27-29, this was assumed to hold only at the level of Planck's length and time. These ideas have now been developed into attempts to build a theory of quantum gravity (see e.g. Ref. 30 and references therein), whose preferential domain of application would be the very early universe.
Geometry and Microphysics
In spite of the advantages one may find in a space-time theory, the several unsuccessful attempts to unify gravitation and electromagnetism from a geometrical approach based on curvature and/or torsion (see e.g. Ref. 31) finally convinced physicists that such an approach has to be given up. The parallel success of quantum gauge theories led to the hope that unification may rather be reached only from the quantized field-particles approach, and that gravitation itself should be quantized in the end. However, among the various causes of the failure of previous geometrical attempts, two may be pointed out in the light of the hereabove remarks:
(i) the observed properties of the quantum world cannot be reproduced by Riemannian geometry;
(ii) the space-time approach cannot be based on particular fields, but on those properties of matter which are universal. It is thus clear that any new insights about the nature of the microphysical space-time may only be gained provided new concepts are introduced.
In this book we shall review the principles and the first results of a new attempt at reconsidering the conclusion that a geometrical approach of the quantum properties of microphysics is impossible. We suggest possible ways towards the construction of a spatio-temporal theory of the microphysical world, basing ourselves on the concept of fractal space-time in connection with the suggestion of an extension of the principle of relativity. The crucial new ingredient of our approach with respect to present standard physics (classical and quantum) is that we assume that space-time is non-differentiable. Moreover we shall demonstrate (Sec. 3.10) that non-differentiability implies an explicit dependence of space-time on scale.
In this quest, our main lead will be Einstein's principle of relativity. But we shall take here relativity as a general method of thinking, rather than as a particular theory. In a relativistic approach to physics, one tries to analyze what, in the expression of physical laws, depends on the particular reference system used, and which properties are independent of it. We shall show that the principle of relativity applies not only to motion, but also to scale transformations, once the resolution of measurements is defined as a state of the coordinate system.
2.2. The Need for a New Extension of Principle of Relativity.
Prior to setting the principle of relativity, there is the definition of coordinate systems and of the possible transformations between these systems. Indeed this principle is a statement about the universality of the laws of physics, whatever the system of coordinates in which they are expressed. So let us try to analyse further what we mean by a system of coordinates. Physics is, above all, a science based on measurements. Its laws apply not to objects by themselves, but rather to the numerical results of measurements which have been or may be performed upon these objects. So the definition of coordinate systems should include all the relevant information which is necessary to describe these results and to relate them in terms of physical laws.
It is an experimental fact that four numbers are necessary and sufficient to locate an event (i.e., a position and an instant): space-time is of topological dimension 4. This operation of location of an event is found to have the following properties:
(i) It cannot be made in an absolute way. This means that an event can be located only with respect to another event, never to some absolute position or instant. What are measured are always space intervals and time intervals. This relativity of events implies that coordinate systems must be firstly characterized by the setting of an origin, O.
(ii) Then one needs to define the axes of the coordinate system. They may be rectilinear, but more generally curvilinear. This means that space-time is covered by a continuous grid or lattice of lines (i.e., of topological dimension 1). In present physics, this curvilinear coordinate system is also assumed to be differentiable.
(iii) We want to characterize by numerical values the position and instant of a second event with respect to O. However length and time intervals are themselves relative quantities: there is indeed no absolute scale in Nature. This second relativity, which we shall call "relativity of scales", is currently translated by the need to use some units for measuring length and time intervals. But we shall argue in the following that its consequences for laws of physics may be far more profound.
(iv) A last property of space-time coordinate measurements (and of any measurement) is that they are always made with some finite resolution. We claim that resolution should be included in the definition of coordinate systems.32,33 Being itself a length or time interval, it is subjected to the relativity of scales. This resolution corresponds to the minimal unit which may be used when characterizing the length or time interval by a final number: e.g., if the resolution of a rod is 1 mm, it would have no physical meaning to express a result in Å. This resolution sometimes corresponds to the precision of the measuring apparatus: it may then eventually be improved, this corresponding to an improved precision of the result in classical physics. But it may also correspond to a physical limitation. For example it is probable that the distance from the Sun to the Earth would never be measured with a precision of 1 Å: this would have no physical meaning. And last but not least, resolution of the measurement apparatus plays in quantum physics a completely new role with respect to the classical, since the results of measurements become dependent on it, as a consequence of Heisenberg's relations.
It is well-known that any set of physical data takes its complete sense only when it is accompanied by the measurement errors or uncertainties, and more generally by the resolution characterizing the system under consideration. Complete information about position and time measurement results is obtained when not only space-time coordinates (t, x, y, z), but also resolutions (Dt, Dx, Dy, Dz) are given. Though this analysis already plays a central part in the theory of measurement and in the interpretation of quantum mechanics, one may remark however that its consequences for the nature of space-time itself have still not been drawn: we suggest that this comes from the fact that, up to now, resolutions have never appeared explicitly in the definition of coordinate systems, while, as shown hereabove, they are explicitly related to the information which is relevant for our understanding of the meaning of actual measurements.
Once the properties of coordinate systems defined, the next task is to describe the possible transformations that are acceptable between these systems. These transformations change the various quantities which define the state of coordinate systems, i.e., following the above analysis: origin, axes, units and resolution.
First consider changes of origin. The invariance of physical laws under static changes of the origin of coordinates systems is translated in terms of homogeneity of space and uniformity of time (more generally, homogeneity of space-time). This is the basis, under Noether's theorem, for the conservation of momentum and energy. So the very existence of energy-momentum as a fundamental conservative quantity (which is itself identified as the charge for gravitation in general relativity) relies on the first relativity, that of position and instants. Velocity-dependent changes of origin may also be considered in space: this leads to Galileo's relativity of inertial motion. But they may also be subsequently included into static rotations in space-time, so this leads us to the second transformation, that of axes.
Axes transformations first include changes of orientation. The invariance of physical laws under rotations in space corresponds to the isotropy of space and yields the conservation of angular momentum. Including rotations in space-time in the transformations considered allows one to describe the relativity of motion as a relativity of orientations in space-time. This yields the Lorentz transform. Finally Einstein's special theory of relativity accounts, in terms of the Poincaré invariance group, for the full relativity of positions, instants and axis orientation.
Then including any continuous and differentiable transformation yields Einstein's general relativity: the change to curvilinear coordinate systems introduces not only non-inertial motion but also curvature of space-time that manifests itself as gravitation.
On this road, it is clear that if one still wanted to generalize the class of acceptable transformations, one should give up differentiability, and then, as a last possibility, continuity (recall that non-differentiability does not imply discontinuity). Let us call "extended covariance" the covariance of the equation of physics under general continuous transforms, including non-differentiable ones. It is also clear that the achievement of such an extended covariance would imply a profound change in the physico-mathematical tool, since the whole mathematical physics is currently founded on integro-differentiation. In this book, we shall try to convince the reader that such an achievement is indeed necessary for our understanding of the foundation of the laws of Nature, in particular in the microphysical domain.
Let us indeed point out what may be considered as remaining defects of the present state reached by physics, which indicate that the principle of relativity of motion itself needs to be extended. Although it became definitively clear after Mach's and Einstein's analysis that the concept of an absolute space-time was to be given up and superseded by a space-time depending on its material and energetic content, the present quantum theory of microphysics still assumes space-time to be Minkowskian, i.e. absolute. This is at variance with the radically new quantum properties of matter and energy, as compared to the classical ones on which the special and general theories of relativity were founded. In other words, the Minkowskian and Riemannian nature of space-time was deduced from the classical properties of objects. In the quantum domain, we know that all objects have quantum properties (all are subjected to Heisenberg's inequalities). We also know that the structure of space-time must depend on its material and energetic content: how, under these conditions, can a space-time whose content is universally quantal be Minkowskian, i.e. flat and absolute?
An additional remark may be made. The goal of a completely general relativity cannot presently be considered as reached, since it is clear that the methods of the present theory of general relativity do not apply to reference frames which would be swept along in the quantum motion, which is continuous but non-differentiable, as discovered by Feynman.34,35 This non-differentiability of virtual and real quantum paths is one of the key points to our own approach. We shall at length come back on it, showing in particular that it can be described in terms of Brownian motion-like fractal properties.36,33
Basing ourselves on these considerations, we have suggested that the principle of relativity still needs to be extended.32,33 Our concept of space-time has evolved from the Galilean independent space and time, to the Minkowskian absolute space-time, then to the Riemannian relative space-time of Einstein's theory. If one wants to include the non-differentiable fractal quantum motion into those described by a theory of relativity, a radically new geometrical structure of space-time must be introduced. Our suggestion is that the quantum space-time is relative and fractal,33 i.e., divergent with decreasing scale (we shall adopt this definition of the word "fractal" here; see Mandelbrot37,38 for other definitions). We shall indeed demonstrate (see Sec. 3.10) that continuity and non-differentiability implies scale divergence. The same conclusion concerning the fractal stucture of space-time will be reached in the next Section, by basing ourselves on the relativity of all scales in Nature.
In our approach, throughout the present essay, we assume space-time to remain a continuum, even if it is no longer assumed to be differentiable. An ultimate choice for physics would be to give up the hypothesis of continuity itself. Some attempts to introduce discontinuous space-times have been made.39,27-29 In this respect let us quote one of these attempts, Moulin's "arithmetic relators", which are defined using purely integer numbers.40 Arithmetic relators are quadratic cellular automata which include internal variables and environment variables. They have proved to be efficient for providing structures, in particular biological ones.41 Such an ability of making structures emerge from very few conditions is reminiscent of "mappings" often used in the study of dynamical chaos. In particular, arithmetic relators yield a hierarchisation, i.e., the various structures appear at different levels of imbrication.
We shall adopt here a more conservative point of view, by keeping the space-time continuum hypothesis and by including the scale dependence in the fundamental principle themselves.
2.3. Relativity of Scales.
We have considered, in the previous Section, the various transformations of the coordinate systems corresponding to changing the origin and axes. The subsequent state of coordinate systems which may be submitted to a transformation are units. Some attempts at including such a transformation in physical laws have been made, in particular in the framework of the conformal group.42,43 Conformal transformations include, in addition to the Poincaré ones, dilatations and special conformal transformations; both of which may be interpreted as related to changes of units. However, while electromagnetic waves are subjected to the conformal symmetry, this is not the case for matter, so that the conformal symmetry cannot be an exact symmetry of nature. Moreover the choice of the unit is in most situations a purely arbitrary one, which does not describe the conditions of measurement, but only their translation into a number.
Let us nevertheless analyse further the physical meaning of units. Their introduction for measuring lengths and times is made necessary by the relativity of every scales in Nature. When we say that we measure a length, what we actually do is to measure the ratio of the lengths of two bodies. In the same way as the absolute velocity of a body has no physical meaning, but only the relative velocity of one body with respect to another, as demonstrated by Galileo, the length of a body or the periods of a clock has no physical meaning, but only the ratio of the lengths of two bodies and the ratio of the periods of two clocks.
When we say that a body has a length of 132 cm, we mean that a second body, to which we have arbitrarily attributed a length of 1 cm and which we call the unit, must be dilated 132 times in order to obtain the first body's length. Measurements of length and time intervals always amount, in the end, to dilatations. The tendency for physics to define a unique system of units was certainly a good thing, since this was necessary for a rational comparison of measurement results from different laboratories and countries. However, this means that the length of all bodies are referred to a same unique body and the period of all clocks to a same clock, this giving a false impression of absoluteness: such a method masks the actual relation between lengths of all bodies in Nature, which is a two-by-two relation.
The fact which allows us to use a unique unit is the simple law of composition of dilatation, r"=r r'. This law is certainly extremely well verified in the classical domain: there is no doubt that a body having a length of 21 m also measures 2100 cm. We however claim that we know nothing about the actual law of dilatation in the two domains of quantum microphysics and cosmology, in which explicit measurements of length and time become impossible. In these two domains length and time intervals are deduced from observation of other variables (energy-momentum at small scale, apparent luminosity and diameter at large scale) and from underlying accepted theories (quantum mechanics and general relativity) which have been constructed assuming implicitly the hereabove standard law of dilatation. (Compare with the status of velocities before the coming of special relativity, when it also seemed self-evident that their law of composition was w = u + v). We shall attack this problem in Chapter 6 (and in Sec. 7.1 for cosmology) and make new proposals based on the principle of scale relativity.
The status of resolutions is related to that of units (in particular they are subject to the relativity of scales), but is actually different and of more far reaching physical importance. Changing the resolution of measurement corresponds to an explicit change of the experimental conditions. Measuring a length with a resolution of 1/10th mm implies the use of a magnifying glass; with 10 mm, we need a microscope; with 0.1 mm, an electron microscope; with 1Å, a tunnel microscope. For even smaller resolutions, the measurements of length become indirect, since the atom sizes are reached and exceeded. When we enter the quantum domain, i.e., for resolutions smaller than the de Broglie length and time of a system (as will be specified afterwards), the physical status of resolutions radically changes. While classically it may be interpreted as precision of measurements (measuring with two different resolutions yields the same result with different precisions), resolution plays a completely different role in microphysics: the results of measurements explicitly depend on the resolution of the apparatus, as indicated by Heisenberg's relations. This is the reason why we think that the introduction of resolution into the description of coordinate systems (as a state of scale) is not trivial, but will instead lead to a genuine theory of scale relativity and the emergence of new physical laws (see Chapter 6).
In present quantum mechanics, the scale dependence is already implicitly
present. However it is explicitly present neither in the axioms
nor in the basic equations. It comes from the interpretation of these equations
thanks to a theory of measurements. Specifically, one writes Schrödinger's
equation, then solve it. This yields a probability amplitude from which
one deduces the probability density; then one may compute the dispersion
of the variable considered, and Born's statistical interpretation of quantum
mechanics ensures that this will give us the standard error of a statistical
ensemble of values resulting from several measurements of this variable.
By extension, this dispersion may also be interpreted as the resolution
of the measurements: e.g., if one makes position measurements with a resolution
Dx, one expects a subsequent dispersion
in the values of the momentum given by Heisenberg's relation sp
~ h/Dx.
However one may require that a complete physical theory includes in its equations the whole set of physical information yielded by experiment. In other words, it may be demanded that a theory of measurement, instead of being externally added to a given theory, becomes an integral part of it.
Such a requirement of explicit expression of the measurement resolutions in the equations of physics begins to be fulfilled in present physics, even though the interpretation is different from ours. Let us quote two approaches where scale-dependent equations are actually written.
One of these domains is that of the theory of measurement in quantum mechanics, concerning in particular the problem of the so-called reduction of the wave-packet (i.e., sudden collapse of the state vector caused by a measurement). This problem, which underlies that of the quantum-classical dualism (where is the transition from quantum to classical; are classical laws approximations of the quantum ones...?), has recently known a resurgence of interest (see Refs. 44 and 45 and references therein). The basic idea of these works is that reduction of the wave packets originates from an interaction of a quantum system with the environment. This interaction is described by a master equation which is explicitly resolution-dependent, so that the transition from quantum to classical behaviour is found to depend directly on resolution in terms of a decoherence time scale44
tD ~ (lT/Dx)2 ,
where lT is the thermal de Broglie length of the system. We shall come back to this approach in Sec. 5.7.
A second domain where explicitly scale-dependent equations have been introduced is the renormalization group approach.46-48 First introduced in quantum electrodynamics as the group of transformation between the various ways to renormalize the theoretical divergences, it became under Wilson's influence a general method of description of problems involving multiple scales of length.48-50 In the renormalization group approach, one writes differential equations describing the infinitesimal variation of physical quantities (fields, couplings) under an infinitesimal variation of scale. The renormalization group will play a leading part in our approach. We shall indeed demonstrate that the renormalization group equations (i) can be interpreted as the simplest lowest order differential equations describing the measure on fractal geometry; (ii) are for scale laws the equivalent of Galileo's group for motion laws. As a consequence we shall propose (in Chapter 6) a generalization of its structure aimed at making it consistent with the principle of scale relativity, which is stated above.
Our first proposal for implementing the idea of scale relativity was to extend the notion of reference system by defining "supersystems" of coordinates which contain not only the usual coordinates but also spatio-temporal resolutions, i.e., (t,x,y,z;Dt,Dx,Dy,Dz).33 The axes of such a reference supersystem would be endowed with a thickness: this corresponds, indeed, to actual measurements. Then we proposed an extension of the principle of relativity, according to which the laws of nature should apply to any coordinate supersystem. In other words, not only general (motion) covariance is needed, but also scale covariance.
Consider now the fundamental behaviour of the quantum world in the light of these ideas. Recall our assumption that when the physicist finds universal properties for physical objects, these properties may be attributed to the nature of space-time itself. This analysis applies particularly well to some of the quantum properties, accounting for their universal character: de Broglie's51 and Heisenberg's52 relations.
Let us recall indeed that the wave-particle duality is postulated to apply to any physical system, and that the Heisenberg relations are consequences of the basic formalism of quantum mechanics (Sec. 2.1). The existence of a minimal value for the product Dx.Dp is a universal law of nature. Such a law, in spite of its universality, is considered in the current quantum theory as a property of the quantum objects themselves (it becomes a property of the measurement process because measurement apparatus are in part quantum and precisely because it is universal). But it is remarkable that it may be established without any hint to any particular effective measurement (recall that it arises from the requirement that the momentum and position wave functions are reciprocal Fourier transforms). So we shall assume that the dependence of physics on resolution pre-exists any measurement and that actual measurements do nothing but reveal to us this universal property of nature: then a natural achievement of the principle of scale relativity is to attribute this universal property of scale dependence to space-time itself.
By such a route, we finally arrive at the same conclusion as that at the end of the previous Section, but this conclusion is now reached by basing ourselves on the principle of scale relativity rather than on the extension of the principle of motion relativity to non-differentiable motion. Namely, the quantum space-time is scale-divergent, according to Heisenberg's relations, i.e., by our adopted definition (see Sec. 2.2 and Chapter 3), fractal. This idea will be more fully developed in Chapters 4 and 5.
However this first formulation of the principle of scale relativity33 is not fully satisfactory. It does not incorporate the complete analysis of the relativity of scales (see above), and treats resolutions on equal footing with space-time variables. However, we have shown that resolutions are more accurately described as a relative state of scale of the coordinate system, in the same way as velocity describes its state of motion. So, in parallel with Einstein's formulation of the principle of motion relativity, we shall finally set the principle of scale relativity in the form53
"The laws of physics must apply to coordinate systems whatever their state of scale."
The full principle of relativity will then require validity of the laws of physics in any coordinate system, whatever its state of motion and of scale. This is completed by a principle of scale covariance (in addition to motion covariance):
"The equations of physics keep the same form (are covariant) under any transformation of scale (i.e. contractions and dilatations)."
We shall see in Chapter 6 that in this form the principles of scale relativity and scale covariance imply a profound modification of the structure of space-time at very small scales: we find that there appears a universal, unpassable, limiting scale in Nature, which is invariant under dilatation, and plays for scale laws a role quite similar to that played by the velocity of light for motion laws (i.e., the limiting scale is neither a cut-off, nor a quantization, nor a discontinuity of space-time). Such a modification has observable consequences at presently accessible energies, which may be expressed in terms of `scale-relativistic' corrections.
2.4. On the Nature of Quantum Space-Time.
All the hereabove arguments indicate that one must give up the absolute Minkowskian space-time postulated in the current quantum theory, and replace it by a space-time which is relative to its material and energetic content and explicitly dependent on scale. Three mathematical methods may be considered to achieve such a program. The first one is geometric: the concept of fractal37,38 refers to objects or sets which are indeed scale-dependent. It must be generalized to that of fractal space,37 while the concept of scale invariance must be extended to that of scale covariance. One may also look for an algebraic tool: the renormalization group is thus very well adapted, but must also be generalized in order to satisfy scale covariance. A third method (which will not be considered here) could be to work in the framework of the conformal group, owing to the fact that it already contains dilatations in its transformations. As a consequence, we expect space-time to be described by a metric element based on generalized, explicitly scale-dependent, metric potentials gmn = gmn(t,x,y,z;Dt,Dx,Dy,Dz).33
Let us specify the physical meaning of this proposal. The concept of space-time allows one to think of all the positions and instants taken together as a whole. Space-time may be viewed as the set of all events and of the transformations between them. But to the set of all events, xm = - infinity to +infinity, (m = 0 to 3), we add the set of all possible resolutions, ln(Dxm) = - infinity to +infinity. Let us call this set "zoom". Hence the geometrical frame in which it will be attempted to work is, strictly speaking, a "zoom-space-time". This means that geometrical structures may be looked for, not only in space-time, but also in the zoom dimension (see Chapter 4). However, as remarked in Sec. 2.3, resolutions and space-time variables do not play an identical role. The "space-time-zoom" is equivalent to phase space rather than an extension of space-time.
The important point to be understood by the reader, since it underlies our whole methods and results, is that we call for a profound change of mentality in the physical approach to the problem of scales. One must give up the "reductionist" view of perfect points whose small scale organization would give rise to the large scale one. One must even go beyond the view of a physics where several particular scales are relevant. We claim that a genuine physics of scale can be constructed only in a frame of thought where all scales in Nature would be simultaneously considered, i.e., when placing ourselves in a continuum of scales. In such a perspective, the standard coordinates themselves lose their physical meaning, and should be replaced by fractal coordinates which are explicitly scale dependent, X=X(s,e) (see Chapters 3 and 5).
The geometrical properties and structures of the microphysical space-time remain to be built in details. As stated above, this book reviews an approach of this problem where it is proposed that, on account of its inferred dependence (and divergence) on resolution, one of the main properties of such a geometry would be its fractal character. We recall that we have previously proposed54 that the concept of fractal should be applied not only to sets or objects embedded in Euclidean space, but to a whole space (more generally space-time) considered in an intrinsic way, i.e., for which curvilinear coordinates, metrics elements, geodesics etc... should be defined. Such an approach may be related to Le Méhauté's,56 who was able to describe new electromagnetic properties arising in fractal media, by using the mathematical tool of non-integer integro-differentiation.
We indeed think that the concept of fractal space-time allows one to revise the conclusion that the quantum mechanical behaviour cannot be derived from a geometrical theory. Several mathematical properties of fractals go in the right direction, e.g.:
* One of the main characteristics of the fractal geometry is its dependence on resolution. Thus it offers a natural way of actualization of the hereabove suggested extension of the principle of relativity, by the use of a spatio-temporal description.
* It was realized by Feynman34,35 that a particle path in quantum mechanics may be described as a continuous and non-differentiable curve, while non-differentiability is one of the properties of fractals. More precisely, a particle trajectory (of topological dimension 1) in nonrelativistic quantum mechanics may be characterized by a fractal dimension 2 when the resolution becomes smaller than its de Broglie length (see Ref. 36 and Chapter 4). This result, being derived from the Heisenberg relation and equivalent to it (see hereafter), is of a universal character. So, in the same way that general relativity attributes to space-time the universal property of curvature of trajectories, our suggestion is to attribute to quantum space-time the universal property of fractalization owned by quantum mechanical trajectories. The implications of this proposal will be specified throughout this book.
* Infinite numbers arise naturally on fractals, and the occurence of infinite quantities is one of the difficulties of current quantum physics. It is remarkable that the infinities which appeared in quantum electrodynamics precisely concern physical quantities like masses (i.e., self-energy) or charges, which are fundamental invariants built from space-time and quantum phase symmetries. One may wonder whether the need for renormalization comes from the lack of account of the irreducible space-time infinities which would be a part of the nature of a fractal space-time.
* Extending the general relativistic approach, the particles in a fractal space-time are expected to follow the "geodesical" lines. But the absence of derivative, the folding and the infinite number of obstacles at all scales due to the fractal structure allow one to infer that an infinity of geodesics will exist between any two points, so that only statistical predictions will be allowed (see Sec. 5.5).
Additional examples of the adequation of fractals and quantum mechanical properties will be reviewed in this book. We are led throughout this work by the postulate that microphysical space-time is a self-avoiding fractal continuum of topological dimension 4. Fractal 4- coordinates are assumed to be defined on this fractal space-time. (Some ways to deal with their infinite character and with their non-differentiability are proposed in Chapter 3). These fractal coordinates correspond to the ideal case of infinite resolution, Dxm = 0. Then the various supersystems of coordinates which correspond to finite resolution will be obtained by smoothing them with "4-balls" (Dt,Dx,Dy,Dz). The classical coordinates (which are independent of resolutions) result from the same smoothing process, but with balls larger than some transitional values lm, corresponding to the fractal/nonfractal transition, which we identify with the quantum/classical transition. Note also that the physical being to be used in order to fit with the "zoom-space-time" idea is not only the fractal itself (i.e. the final result of a fractalization process), but mainly the set of all its approximations for all possible values of space-time resolution. Some of its other properties will gradually emerge, while attempts will be made to express the main quantum mechanics results in terms of geometrical fractal structures.
In particular, our application of the principle of scale relativity to the question of the fractal dimension of quantum paths leads us to the conclusion that the constant fractal dimension D=2 obtained from standard quantum physics is only a "large" scale approximation. This Brownian-motion like fractal dimension (see Sec. 5.6) may also be interpreted in terms of a constant anomalous dimension d=1. But this constant value corresponds to "Galilean" scale laws, while the requirement of scale covariance leads us to the conclusion that the correct renormalization group for space-time must be a Lorentz group (Chapter 6).
Already the new structure of space-time revealed by special motion relativity at the beginning of the century underlies in an inescapable way the Riemannian structure of Einstein's general relativity: Space-time is locally Minkowskian, so that all attempts at constructing a Riemannian theory of gravitation were condemned to fail in the absence of special relativity constraints. In the same way (assuming that the whole approach is correct) if a full theory of scale relativity is to be developed one day in terms of fractal space-time, we think that such a theory will be forced to incorporate in its description the new structure of space-time which is described in Chapter 6: a space-time where the perfect zero point has disappeared from concepts having physical meanings, whose fractal dimension is not constant but scale-dependent and whose local invariance group is Lorentz's, for motion as well as scale transformations.
2 References
1. Einstein, A., 1905, Annalen der Physik 17, 891. English translation in "The principle of relativity", (Dover publications), p. 37.
2. Poincaré, H., 1905, C. R. Acad. Sci. Paris 140, 1504.
3. Einstein, A., 1916, Annalen der Physik 49, 769. English translation in "The principle of relativity", (Dover publications), p. 111.
4. Schrödinger, E. von, 1925, Ann. Phys. 79, 361.
5. Heisenberg, W., 1925, Zeitschrift für Physics 33, 879.
6. Dirac, P.A.M., 1928, Proc. Roy. Soc. (London) A117, 610.
7. Salam, A., in Elementary Particle Physics (Almqvist & Wiksell, Stockholm, 1968).
8. Weinberg, S., 1967, Phys. Rev. Lett. 19, 1264.
9. Glashow, S., 1961, Nucl. Phys. 22, 579.
10. Dirac, P.A.M., in "Unification of fundamental forces", 1988 Dirac Memorial Lecture, (Cambridge University Press, 1990), p.125.
11. Feynman, R.P., 1949, Phys. Rev. 76, 769.
12. Schwinger, J., 1948, Phys. Rev. 74, 1439.
13. Tomonaga, S., 1946, Prog. Theor. Phys. 1, 27.
14. Thurston, W.P., & Weeks, J.R., 1984, Sci. Am. 251, 94.
15. Pais, A., `Subtle is the Lord', the Science and Life of Albert Einstein (Oxford University Press, 1982).
16. Lorenz, E.N., 1963, J. Atmos. Sci. 20, 130.
17. Ruelle, D., & Takens, F., 1971, Commun. Math. Phys. 20, 167.
18. Eckmann, J.P., & Ruelle, D., 1985, Rev. Mod. Phys. 57, 617.
19. Levy-Leblond, J.M., 1976, Am. J. Phys. 44, 271.
20. Minkowski, H., 1908, Address delivered at the 80th assembly of german natural scientists and physicians, Cologne, English translation in "The principle of relativity", (Dover publications), p. 75.
21. Einstein, A., 1911, Annalen der Physik 35 , 898. English translation in "The principle of relativity", (Dover publications), p. 99.
22. Misner, C.W., Thorne, K.S., & Wheeler, J.A., Gravitation (Freeman, San Francisco, 1973).
23. Bjorken, J.D., & Drell, S.D., Relativistic Quantum Mechanics (Mac Graw Hill, 1964).
24. Schiff, L.I., Quantum Mechanics (Mac Graw Hill, 1968).
25. Feynman, R.P., Leighton, R., & Sands, M., The Feynman Lectures on Physics (Addison-Wesley, 1964).
26. Balian, R., 1989, Am. J. Phys. 57, 1019.
27. Fuller, R.W., & Wheeler, J.A., 1962, Phys. Rev. 128, 919.
28. Wheeler, J.A., in Relativity Groups and Topology (Gordon & Breach, New York, 1964).
29. Hawking, S., 1978, Nucl. Phys. B144, 349.
30. Isham, C.J., in 11th International conference on General Relativity and Gravitation, M. MacCallum ed., (Cambridge University Press, 1986) p.99.
31. Lichnerowicz, A., Théories Relativistes de la Gravitation et de l'Electromagnétisme (Masson, Paris, 1955).
32. Nottale, L., 1988, C. R. Acad. Sci. Paris 306,341.
33. Nottale, L., 1989, Int. J. Mod. Phys. A4, 5047.
34. Feynman, R.P., 1948, Rev. Mod. Phys. 20, 367.
35. Feynman, R.P., & Hibbs, A.R., Quantum Mechanics and Path Integrals (MacGraw-Hill, 1965).
36. Abbott, L.F., & Wise, M.B., 1981, Am. J. Phys. 49, 37.
37. Mandelbrot, B., Les Objets Fractals (Flammarion, Paris, 1975).
38. Mandelbrot, B., The Fractal Geometry of Nature (Freeman, San Francisco, 1982).
39. Brightwell, G., & Gregory, R., 1991, Phys. Rev. Lett. 66, 260.
40. Moulin, Th.,1991, International Review of Systemic, in the press
41. Vallet, Cl., Moulin, Th., Le Guyader, H., & Lafrenière, L., 1976, in VIIIth International Congress of Cybernetics, Namur (Belgium), p.187.
42. Barut, A.O., & Haugen, R.B., 1972, Ann. Phys. 71, 519.
43. Callan, C.G., Coleman, S., & Jackiw, R., 1970, Ann. Phys. 59, 42.
44. Unruh, W.G., & Zurek, W.H., 1989, Phys. Rev. D40, 1071.
45. Zurek, W.H., 1991, Physics Today vol. 44, ndeg.10, p.36.
46. Gell-Mann, M., & Low, F.E., 1954, Phys. Rev. 95, 1300.
47. Stueckelberg, E., & Peterman, A., 1953, Helv. Phys. Acta 26, 499.
48. Wilson, K.G., 1975, Rev. Mod. Phys. 47, 774.
49. Wilson, K.G., 1983, Rev. Mod. Phys. 55, 583.
50. de Gennes, P.G., Scaling concepts in Polymer Physics (Ithaca, Cornell Univ. Press, 1979).
51. Broglie, L. de, 1923,C.R. Acad. Sci. Paris 177, 517.
52. Heisenberg, W., 1927, Z. Physik 43, 172.
53. Nottale, L., 1992, Int. J. Mod. Phys. A, in the press.
54. Nottale, L., & Schneider, J., 1984, J. Math. Phys. 25, 1296.
55. Weinberg, S., Gravitation and Cosmology (Wiley & Sons, New York, 1972).
56. Le Méhauté, A., Les Géométries Fractales (Hermès, Paris, 1990).